| Atomic Theory |
| 1. Rutherford's Model of the atom | 2. The Bohr Atom |
Rutherford's Model of the atom:
Rutherford proposed the nuclear model of the atom on the basis of his experimental results of the scattering of alpha-particles by the atoms. In his experiment alpha-particles emitted with speeds of about 2 x 107 m/s struck a thin gold foil several thousand atomic layers thick. Most of the alpha-particles pass undeflected through the foil, but some were scattered at some angle. According to Rutherford's model of the atom, almost the entire mass and the total positive charge of the atom are confined within a very small part at the center of the atom. This part is known as the nucleus of the atom and has a radius less than 10-12 cm which is small compared to the radius of the atom (approx. 10-8 cm). The electron in the atom revolves in orbits around this central core. The radii of these orbits determine the atomic radii.
Please on the link below to see a simulation of Rutherford's alpha-particle scattering:
Simulation of Rutherford's alpha-particle scattering
Drawback of Rutherford's model:
According to electromagnetic theory an accelerated particle loses energy by the emission of electromagnetic radiation. So, the electrons revolving in this atomic orbits will loose energy by emitting e.m. radiation and thus eventually will collapse onto the positively charged nucleus of the atom. Thus there cannot be any stable atom! This is the main drawback of Rutherford's simple picture of the atom.
Bohr solved this using quantum mechanical concepts.
The Bohr atom:

Bohr's theory of atom is based on three fundamental postulates:
(a) The electron can revolve in a number of specified circular orbits, known as stationary orbits, around the central positively charged nucleus. In each stationary orbit, the electron possesses an orbital angular momentum
p = nh/(2p) = n hbar,
where n=1,2,3,... is an integer known as the quantum number and hbar = h/2p (h is the Planck's constant).
(b) In a stationary orbit the electron does not radiate electromagnetic energy.
(c) When an electron makes a transition from an initial stationary orbit of higher energy Ei to a final stationary orbit of lower energy Ef, it emits e.m. radiation of energy
hf = Ei - Ef ,
where f is the frequency of radiation. The above condition is known as Bohr's frequency condition.
If the energy Ei of the initial orbit is less than the energy Ef of the final orbit, then a transition between them will be possible only if the electron absorbs energy from an incident radiation of energy hf = Ei - Ef .
|
Radius and Energy of the Bohr orbit:
Consider the mass and charge of the electron to be m and -e and the nuclear charge is +Ze. Also we assume that the nuclear mass (M) is infinitely large compared to the electron mass. Equating the electrostatic attraction between the nucleus and the electron to the centrifugal force we get:
mv2/r = Ze2/r2. Eq. (1)
The angular velocity w of the revolving electron is related to the velocity as v = w r. We can rewrite Bohr's quantum condition as follows:
p = mw2r = mvr = n hbar. Eq. (2)
r is the radius of the electron orbit. From Eqs. (1) and (2) we get -
r = rn = n2 hbar2/(mZe2), v = vn = n hbar/mrn = Ze2/(n hbar).
The kinetic and potential energies of the electrons are:
T = mv2/2 = Ze2/2r, V = -Ze2/r.
The total energy is,
E = T + V = -Ze2/r,
=> En = -mZ2 e4/(2n2 hbar2). Eq. (3)
rn is the radius of the n-th orbit and En is the total energy of the electron in this orbit.
Eq. (3) gives a set of discrete energy levels for the electron, the energy being the lowest for n=1 which is known as the ground state of the electron.
The energy emitted in the form of radiation when an electron makes a transition from an orbit with n = ni to an orbit with n = nf is given by (ni > nf)
hf = Ei - Ef = (mZ2e4/2 hbar2)(1/nf2 - 1/ni2). Eq. (4)
The energy needed to ionize an atom (nf -> infinity) which is initially in the ground state (ni = 1) is (energy is absorbed in this case)
I = mZ2 e4/ (2hbar2).
For hydrogen (Z=1), this gives I ~ 13.6 eV.
Defining f'= f/c = 1/l as the wave number, we get from Eq. (4) for the transition ni -> nf,
f' = (mZ2e4/4pc hbar2)( 1/nf2 - 1/ni2) = R Z2 (1/nf2 - 1/ni2), Eq. (5)
for the emission of radiation (nf < ni). For absorption ni and nf are interchanged in the above formula. The transitions for the emission and absorption of radiation are shown in the Figure below.
Note that R = me4/4 p c hbar2 = 109, 737 cm-1 is a universal constant and is known as the Rydberg constant.
For a given value of the final state quantum number nf, transitions from different initial states give rise to lines of a particular spectral series.
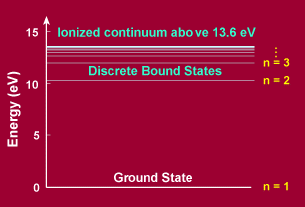
In the case of hydrogen (Z=1) a number of these series have been identified:
Lyman series: nf= 1, ni=2, 3, 4, ....
f' = RH (1 - 1/ni2 )
Balmer series: nf= 2, ni=3, 4, 5, ....
f' = RH (1/4 - 1/ni2 )
Paschen series: nf= 3, ni=4, 5, 6,....
f' = RH (1/9 - 1/ni2 )
Brackett series: nf= 4, ni=5, 6, 7, ....
f' = RH (1/16 - 1/ni2 )
The different spectral series of hydrogen experimentally measured are in excellent agreement with Boh'r theory. The experimental verification of Bohr's theory constitues another triumph of the quantum hypothesis.
To view the energy levels of the Hydrogen atom please below -
Please on the hyperlink below to see a simulation of the Bohr atom:
Some cool sites on the Bohr atom:
© Kingshuk Majumdar (2000)