|
GVSU
REU SUMMER
2014 THE
GEOMETRY OF THE HAUSDORFF METRIC Steven Schlicker |

A
Polygonal Chain Generating a Previously Unknown Integer Sequence
My Background
I started conducting research with undergraduate students in 1990 with a project involving fractal geometry and regular polygons. I continued this type or research when I came to Grand Valley, ultimately deciding to apply to the National Science Foundation to form a Research Experiences for Undergraduates (REU) site at GVSU. We hosted our first REU site in 1999 and our program has been running strong ever since. My current interests are in the geometry the Hausdorff metric imposes on the space of compact sets, and I have been engaged in this area since 2001. My groups have published several papers (listed in the references) and presented their work at local, regional, and national conferences. The sections below provide a bit of information about this project for summer 2014, and I look forward to continuing this research.
What Will This Research Involve?
I study the geometry of the
Hausdorff metric. You are probably familiar with standard Euclidean geometry
where we study relationships between points, lines, and line segments. The
geometry we will explore in this summer REU is quite different – the “points”
are objects (like your coffee cup or I-Pad) and the Hausdorff metric provides a
way for us to measure the distance between these objects. This distance then
imposes a geometry on the space of objects, and it is that geometry that we
will study.
We have learned a lot about this
geometry in past REUs. For example, we have completely classified circles in
this geometry, have made connections to number theory (including discovering an
infinite three-parameter family of integer sequences – connected to the figure
at the top of this page), found that lines in this geometry can just stop, and
proved fascinating facts about the primes 19 and 37. Most recently, we have
been investigating the concept of orthogonality in this space with a goal of
trying to define a trigonometry of sets.
Potential Projects for Summer 2014
·
Considering different notions of
orthogonality. In our last REU we learned much about Pythagorean orthogonality
of sets (the picture at the bottom of this page shows one Pythagorean tripe of
sets), but the ultimate results do not lead us completely to our goal of
defining a trigonometry of sets. There may be other notions of orthogonality (e.g.,
minimizing some distances) that provide better results. We would study different
ways of defining orthogonality, compare and contrast them, and then decide with
which version to proceed.
·
Pursue the concept of Pythagorean
orthogonality in different contexts. For example, there is a way to define a
metric on groups, and so we could investigate what subgroups of certain types
of groups form Pythagorean triples. There may be other interesting sets on
which we could do the same.
·
Even though Pythagorean
orthogonality doesn’t give us everything we want, we might still be able to
define a restricted type of trigonometry of compact sets and investigate its
properties.
·
I’m open to other topics as well,
e.g., searching for other unknown integer sequences within this geometry.
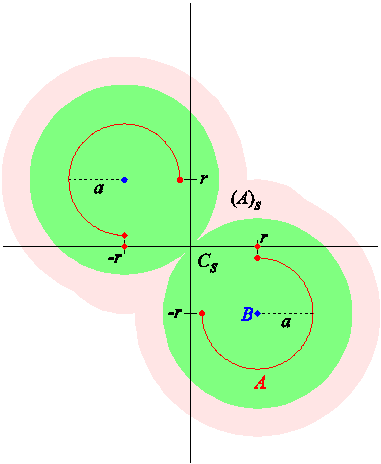
Sets
A, B, and Cs form
a Pythagorean triple
Desirable Experiences for Applicants
Applicants should be willing to work collaborative
with peers and faculty, but also be capable of independent work. An interest in
geometry is important – many of the arguments made in our research involve
basic properties of Euclidean lines, segments, angles, and circles – but it is
not necessary to have more than a basic familiarity with Euclidean
geometry. Most desirable is a positive
attitude toward geometric ideas and the willingness to just muck around with
sets.
What
this Project is NOT...
Although
the Hausdorff metric has many real life applications, I know almost nothing
about them and they are not related to this research. This is a project in PURE MATHEMATICS.
How
to Apply
For
application information and instructions, please visit the GVSU Summer Mathematics REU home
page.
References
·
Missing
edge coverings of bipartite graphs and the geometry of the Hausdorff metric,
Katrina Honigs*. Journal
of Geometry, April 2013, Volume
104, Issue 1,
107-125.
·
A
Missing Prime Configuration in the Hausdorff Metric
Geometry, Chantel Blackburn*,
Kristina Lund*, Steven Schlicker, Patrick Sigmon*,
and Alex Zupan*.
Journal of Geometry, (2009),
92, Numbers 1-2, 28-59.
·
Polygonal
chain sequences in the space of compact sets, Steven
Schlicker, Lisa Morales* and Dan Schultheis*. Journal of Integer Sequences, Vol. 12
(2009), Article 09.1.7.
·
Fibonacci
sequences in the space of compact sets, Kris Lund*, Steven
Schlicker, and Patrick Sigmon*. Involve, Vol. 1 (2008), No. 2, 197-215,
·
A Singular Introduction to the Hausdorff
Metric Geometry, Dominic Braun*, John Mayberry*, Audrey Malagon,
and Steven Schlicker. Pi Mu Epsilon
Journal, Vol. 12, No. 3, p. 129-138, 2005.
·
When Lines Go Bad In Hyperspace,
Christopher Bay*, Amber Lembcke*, and Steven
Schlicker. Demonstratio Mathematica,
No. 3, Volume XXXVIII (2005), p. 689-701.
·
Nine
entries in the On-Line
Encyclopedia of Integer Sequen ces: A152927,
A152928, A152929, A152930, A152931, A152932, A152933, A152934, A152939.